Kinetic theory of gases
Postulates or assumptions of kinetic theory of gases
1)Every gas is made up of a large number of extremely small particles called molecules. All the molecules of a particular gas are identical in mass and size and differ in these from gas to gas.
2)The molecules of a gas are separated from each other by large distances so that the actual volume of the molecule is negligible as compared to the total volume of the gas.
3)The distance of separation between the molecules are so large that the forces of attraction or repulsion between them are negligible.
4)The force of gravitation on the molecule is also supposed to be negligible.
5)The molecules are supposed to be moving continuously in different direction with different velocities. Hence they keep on colliding with one another as well as on the walls of the containing vessel.
6)The molecules are supposed to be perfectly elastic hard sphere so that no energy is wasted when the molecules collide with one another or with the walls of the vessel. The energy may however be transferred from some molecules to the other on collision.
7)Since the molecules are moving with different velocities, they possess different Kinetic energies. However ,the average kinetic energy of the molecules of a gas is directly proportional to the Absolute temperature of the gas.
8)The molecules are supposed to be moving continuously in different direction with different velocities. Hence, they keep on colliding with one another as well as on the walls of the containing vessel.
Kinetic gas equation
where P= pressure exerted by the gas
V= volume of the gas
m= mass of each molecule of the gas
N= Total number of molecules of the gas present in volume V
c= Root mean square speed of the gas
Kinetic energy and Molecular speeds
In a gas ,the molecules are moving in different directions with different speed colliding with one another as well as the walls of the container. Their individual speeds and hence Kinetic energies keep on changing even at the same temperature. It is found that at a particular temperature, the average kinetic energy of the gas remains constant.
At a particular temperature if n1 molecules have speed v1, n2 molecules have speed v2 ,n3 molecules have speed v3 and so on, then total kinetic energy of the gas at this temperature will be
Ek = ½ m ( n1v12 + n2 v22 + n3 v32+ …..)
where m is the mass of the molecule of the gas.
Dividing by the total number of molecules, average kinetic energy of the gas will be
½ m( n1v12 + n2 v22 + n3 v32+ …./ n1 + n2 +n3 )
( n1v12 + n2 v22 + n3 v32+ …./ n1 + n2 +n3 ) represents the mean of the square of the speeds of different molecules and hence is called mean square speed.Its square root is called root mean square speed.
Root mean square speed may be defined as the square root of the mean of the squares of the speed of different molecules of the gas.
c = ( n1v12 + n2 v22 + n3 v32+ …./ n1 + n2 +n3 )½
From Kinetic gas equation,
PV= 1/3 mnc2
PV=1/3 Mc2
c= (3PV /M)½
Putting M/V =d , we get
c= (3P/d )½
c= ( 3RT/M )½
Relation between average kinetic energy and absolute temperature
From Kinetic gas equation, we have,
PV = 1/3 mnc2
For 1 mole of a gas, we have
PV = 1/3 Mc2
PV = 2/3 × 1/2 Mc2
But 1/2 Mc2 = Ek , total kinetic energy of 1 mole of the gas
Hence, PV = 2/3 Ek
PV = RT for 1 mole of the gas,
RT = 2/3 Ek
Ek = 3/2 RT
Average kinetic energy per molecule ,divide by Avogadro’s number (NA)
Ek avg = 3RT/2NA
Ek avg = 3/2 kT
where k= R/NA
is called Boltzmann constant = 1.38 × 10-23 JK-1
Average Speed
The arithmetic mean of the speeds of different molecules of the gas.If n1 molecules have speed v1 , n2 molecules have speed v2 ,n3 molecules have speed v3 and so on, the average speed will be given by
c avg = (n1 v1 + n2 v2 +n3 v3 +….) / (n1 +n2 +n3 +…)
cavg = (8RT / πM )½
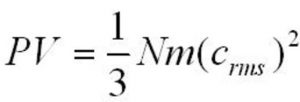
Helpful.