Ideal and Non-Ideal Solution

Ideal and Non-Ideal Solution 

The binary liquid-liquid solution may be classified into two types :

(1) Ideal solutions

(2) Non-ideal solutions

1) Ideal Solutions

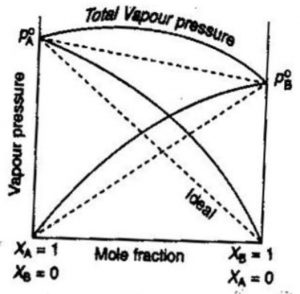
An ideal solution may be defined as the solution which obeys Raoult’s law over the entire range of concentration.

a) Such solutions are formed by mixing two components which are identical in molecular size, in structure and have almost identical intermolecular forces.

b)The intermolecular interactions between the components (A – B attractions) are of same magnitude as the intermolecular interactions in pure components (A- A and B-B attractions).

According to Raoult’s law, the partial vapour pressure of two components of the solution may be given as :

p_A = p_A°x_A

p_B = p_B°x_B

Total pressure p is given by

p= p_A +p_B

p= p_A°x_A + p_B°x_B

The ideal solutions have also the following characteristics: 

1) Heat change on mixing is zero: Since there is no change in magnitude of the attractive forces in the two components present, the heat change on mixing i.e. Δ_mixingH in such solutions must be zero.

2) Volume change on mixing is zero: In ideal solutions, the volume of the solution is the sum of the volumes of the components before mixing i.e. there is no change in volume on mixing or Δ_mixing V is zero.

The solutions generally tends to become ideal when they are dilute.

The characteristics of an ideal solution may be summed up as follows

(i) It must obey Raoult’s law.
(ii) Δ_mixing H should be zero.
(iii) Δ_mixing V should be zero.

Example of Ideal Solutions

(i) Benzene and toluene
(ii) n-hexane and n-heptane
(iii) Bromoethane and iodoethane
(iv) Chlorobenzene and bromobenene

Solutions which obey Raoult’s law are called ideal liquid solution.

Composition in Vapour Phase

The composition of vapour phase in equilibrium with the solution is determined by the partial pressure of the components. If y₁and y₂ are the mole fraction of the two components 1 and 2 respectively in the vapour phase, then according to Dalton’s law of partial pressures:

Partial pressure of a component =Mole fraction of the component x Total pressure

p₁= y₁ p

p₂ =y₂ p

In general, P_i = y_i p _total

Mole fraction of component 1 in vapour phase y₁ = p₁/p

Mole fraction of component 2 in vapour phase, y₂ = p₂ /p

Mole fraction of a component in vapour phase = 

Partial vapour pressure of component / Total Vapour Pressure

2) Non-Ideal Solutions

The solutions which do not obey Raoult’s law over the entire range of concentration are called non-ideal solutions. Therefore, for such solutions

p_A ≠ p_A° x_A

p_B ≠ p_B° x_B

The vapour pressure of such solutions is either higher or lower than that predicted by Raoult’s law.

In non-ideal solutions, there is a noticeable change in volume and heat energy when the two components are mixed.

Most of the solutions are non-ideal because they deviate from ideal behaviour to more or less extent.

Thus, for non-ideal solutions,

a) none of the components obey Raoult’s law over the entire composition range, i.e.

p_A ≠ p_A° x_A and  p_B ≠ p_B° x_B

b) (i) Δ_mixing H is not equal to zero

(ii) Δ_mixing V is not equal to zero

Ideal solution	Non-Ideal solution
The interactions between the components are similar to those in the pure components.	The interaction between the components are different from those of the pure components.
There is no enthalpy change on mixing. ΔmixingH =0	There is no enthalpy change on mixing. ΔmixingH ≠ 0
There is no volume change on mixing. Δmixing V =0	There is no volume change on mixing. Δmixing V ≠ 0
Each component obeys Raoult’s law at all temperatures and concentrations, pA = pA°xA  and pB = pB°xB	Their components do not obey Raoult’s law. They show positive and negative deviations from Raoult’s law. pA ≠ pA° xA and  pB ≠ pB° xB

Types of Non-ideal Solutions

Non-ideal solutions show positive and negative deviations from the ideal behaviour depending upon their nature.

(1)  Non-Ideal solutions showing positive deviations from Raoult’s law

Consider a binary solution of two components A and B.

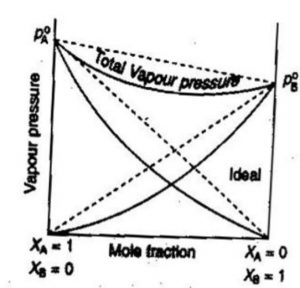
If the A-B interactions in the solutions are weaker than the A-A and B-B interactions in the two liquids forming the solution, then the escaping tendency of A and B types of molecules from the solution becomes more than from pure liquids. As a result, each component of solution has a partial vapour pressure greater than expected on the basis of Raoult’s law. The total vapour pressure will be greater than corresponding vapour pressure expected in case of ideal solution of the same composition. This type of behaviour of solution is described as positive deviations from Raoult’s law. 

p_A >p_A° x_A  and

p_B >p_B° x_B

The total vapour pressure,

p= p_A+ p_B is always greater than (p_A° x_A +  p_B° x_B )

Examples of solutions showing positive deviations are :

(i) Ethyl alcohol and cyclohexane
(ii) Acetone and carbon disulphide
(iii) Benzene and acetone
(iv) Carbon tetrachloride and choloroform
(v) Acetone and ethyl alcohol
(vi) Ethyl alcohol and water.

Explanation for positive deviations

In ethyl alcohol, the molecules are held together due to hydrogen bonding.When cyclohexane is added to ethyl alcohol, the molecules of cyclohexane tend to occupy the spaces between ethyl alcohol molecules. Consequently, some hydrogen bonds in alcohol molecules break and the attractive forces in alcohol molecules are weakened. The escaping tendency of alcohol and cyclohexane molecules from the solution increases. Consequently, the vapour pressure of the solution is greater than the vapour pressure as expected according to Raoult’s law.

(i) Δ_mixingH is positive because energy is required to break A-A or B-B attractive forces.Therefore, dissolution process is endothermic.

(ii)Because of the decrease in the magnitude of intermolecular forces in solutions, the molecules will be loosely held and, therefore, there will be increase in volume on mixing. Thus, Δ_mixingV will be positive.

(iii) Since the dissolution process in endothermic, heating will increase the solubility of such a solution.

(2) Non-Ideal solutions showing negative deviations from Raoult’s law.

In such solutions, the A-B interactions are stronger than the A-A and B-B interactions present in the two liquids forming the solution. Due to stronger A-B interactions, the escaping tendency of A and B types of molecules from the solution becomes less than from pure liquids. Consequently, each component of the solution has a partial vapour pressure less than expected on the basis of Raoult’s law. As a result , the total vapour pressure becomes less than the corresponding vapour pressure expected in case of ideal solution.

p_A < p_A° x_A  and

p_B < p_B° x_B

p= p_A+ p_B is always less than (p_A° x_A +  p_B° x_B )

Examples of Negative Deviation 

(i) Acetone and chloroform
(ii) Chloroform and diethyl ether
(iii) Chloroform and nitric acid
(iv) Acetone and aniline
(v) Water and nitric acid
(vi) Diethyl ether and chloroform.

Explanation for Negative Deviations

When acetone and chloroform are mixed there are new attractive forces due to intermolecular hydrogen bonding. Thus the attractive forces become stronger and the escaping tendency of each liquid from the solution decreases. Therefore, the vapour pressure of the solution is less than that expected for an ideal solution.

(i) Δ_mixingH is negative because energy is released due to increase in attractive forces.Therefore, dissolution process is exothermic and heating the solution will decrease solubility.

(ii)Because of the increase in the magnitude of forces of attraction in solutions, the molecules will be loosely held more tightly. Therefore, there will be decrease in volume on mixing. Thus, Δ_mixingV will be negative.

Difference between Ideal and Non-Ideal solution

Solution having positive deviation	Solution having negative deviation
A-B forces are less than A-A and B-B forces.	A-B forces are more than A-A and B-B forces.
pA >pA° xA  and pB >pB° xB	pA < pA° xA  and pB < pB° xB
ΔmixingH is positive	ΔmixingH is negative
Dissolution is endothermic	Dissolution is exothermic
Heating increases solubility	Heating decreases solubility
ΔmixingV will be positive.	ΔmixingV will be negative.

Azeotropes

a) Some solution exhibit very large positive deviation from Raoult’s law that there is a maximum in the vapour pressure curve which is above the vapour pressure of either pure components.

For one of the intermediate composition, the total vapour pressure of such a solution will be the highest and the boiling point will be the lowest. At this point, the composition of liquid and vapour phase is same and the liquid mixture boils at constant temperature and remains unchanged in composition. Therefore, this liquid mixture distills over as if it is a pure liquid. Solution acquires the property of boiling at constant temperature and remains unchanged in composition.

The solutions (liquid mixtures) which boil at constant temperature and can distil unchanged in composition are called azeotropes or azeotropic mixtures. Thus, the azeotropes distil over as if it were pure liquids. These types of solutions are called minimum boiling azeotropes.

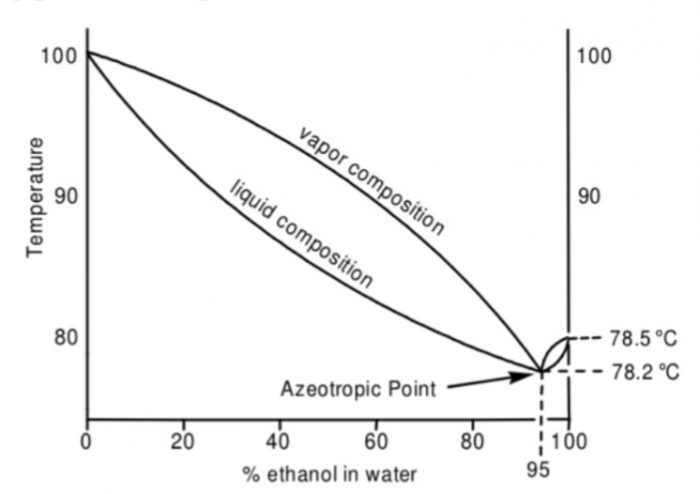
For example: Ethanol and water form minimum boiling azeotrope. It has maximum in the vapour pressure curve and hence a minimum in the boiling point diagram. In this boiling point diagram, we indicate the composition of the vapour phase by the upper curve and composition of the liquid phase by the lower curve.

The boiling point diagram shows an azeotropic composition at x(H₂O)=0.056 and  x(C₂H₅OH) = 0.944 at a temperature.of 351 K (or 78°C) which is lower than that of pure ethanol 351.5 K (or 78.5°C) and water 378 K (or 100°C).

Fractional distillation of solutions lying on either side of this azeotropic composition is capable of separating them into at best, one pure component and a azeotropic mixture having the minimum boiling point.

b) In the case of solutions showing negative deviations, total vapour pressure becomes less than the corresponding ideal solution of same composition. The boiling points of such solutions are increased.

For one of the intermediate composition, the total vapour pressure will be the least and the boiling point will be the highest. At this composition, the solution also boils at constant temperature without a change in composition. This is also called azeotrope.

The solutions which show negative deviations from Raoult’s law are called maximum boiling azeotropes because they have a composition having maximum boiling point

For example: hydrochloric acid and water form maximum boiling point azeotrope at the composition x(H₂O)=0.889 and x (HCl)=0.111 (or 20.2% HCl) which boils at 381.6 K (or 108.6°C) which has higher than that of pure water.

Nitric acid (HNO₃) and water also form maximum boiling azeotrope. The azeotrope has the approximate composition 68% nitric acid and 32% water by mass with a boiling point of 393.5 K.

Thus, the azeotropes are liquid mixtures of definite composition, which boil at constant boiling point. These cannot be separated into pure components by fractional distillation.