Heat Capacity

Heat capacity of a system is defined as the amount of heat required to raise the temperature of the system through 1°C .

If q is the amount of heat supplied to a system and as a result ,if the temperature of the system rises from T₁ and T₂ ,then the heat capacity of the system is given by

C = q / ( T₂ -T₁ )

C= q / Δ T

Since the heat capacity varies with temperature , therefore, the value of C has to be considered over a very narrow temperature range. If  δq is small amount of heat absorbed by a system which raises the temperature of the system by a small amount dT , then the heat capacity of the system will be given by

C= δq /dT

Specific heat capacity , c, of a substance is defined as the amount of heat required to raise the temperature of 1 gram of a substance through 1°C.

Molar heat capacity of a substance is defined as the amount of heat required to raise the temperature of 1 mole of a substance through 1° C.

C_m = C/n

The amount of heat , q , required to raise the temperature from T₁ to T₂ of mass m grams of a sample and having specific heat capacity, c, can be calculated as:

q= m × c × ΔT

q = C × ΔT

Specific heat capacity of water is 1 cal g^-1 K^-1  or 4.18 J g^-1 K^-1.

Types of heat capacity or molar heat capacity

q is not a state function and depend upon the path followed, therefore C is also not a state function.

There are two types of heat capacities :

1)Heat capacity at constant volume (C_v)

2)Heat capacity at constant pressure(C_p)

The heat supplied to a system to raise its temperature through 1° C keeping the volume of the system constant is called heat capacity at constant volume.

 

The heat supplied to a system to raise its temperature through1° C keeping the external pressure constant is called heat capacity at constant pressure.

According to first law of thermodynamics,

δq = dU + PdV

C= δq / dT

C= (dU + PdV) / dT

when the volume is kept constant, dV=0

C_v = ( ∂U / ∂T )_v

For an ideal gas,

C_v = dU / dT

The heat capacity at constant volume may be defined as the rate of change of internal energy with temperature at constant volume.

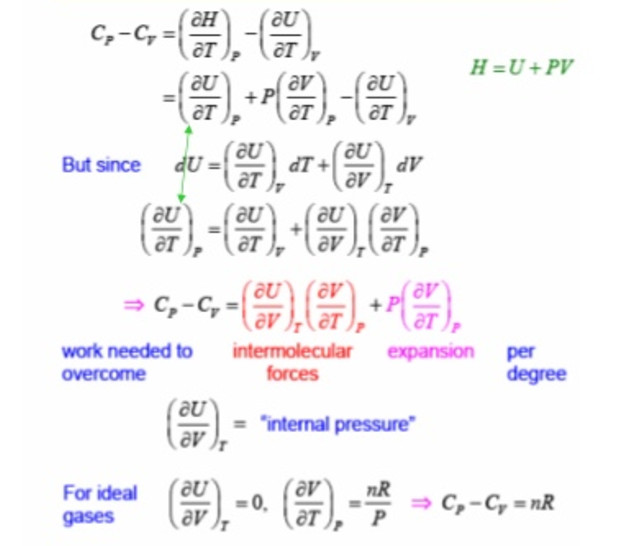
Cp = ( ∂U / ∂T )_p + P ( ∂V / ∂T )_p

H= U + PV

(∂H / ∂T)_p = (∂U / ∂T)_p + P (∂V / ∂T)_p

C_p = (∂H / ∂T)_p

For an ideal gas,

C_p = dH / dT

The heat capacity at constant pressure may be defined as the rate of change of enthalpy with temperature at constant pressure.

Relationship between C_p and C_v

If the volume of the system is kept constant and the heat is added to a system, then no work is done by the system. The heat absorbed by the system is used up completely to increase the internal energy of the system.

If the pressure of the system is kept constant and heat is supplied to the system, then some work of expansion is also done by the system in addition to the increase in internal energy.

If at constant pressure, the temperature of the system is to be raised through the same values as at constant volume, then some extra heat is required for doing work of expansion.Hence C_p > C_v