Variation of Conductivity and Molar Conductivity

Variation of Conductivity and Molar conductivity with conductivity

Electrolytic conductance decreases with increase in  concentration or increases with increase in dilution. This is because conductance of ions is due to the presence of ions in the solution. The greater the number of ions, the greater is the conductance. As with dilution, more ions are produced in solution so conductance also increases on dilution.

Both specific conductance or conductivity and molar conductivity change with concentration of the electrolyte. Conductivity of an electrolyte decreases with the decrease in concentration both for weak and strong electrolytes, whereas molar conductivity increases with decrease in concentration.

Upon dilution, specific conductance or conductivity decreases while molar conductivity increases

Conductivity is the conductance of one centimeter cube of the solution. Upon diluting the solution, the concentration of ions per centimeter cube decreases and therefore, the conductivity decreases.

 

The increase in molar conductivity on dilution is due to the fact that it is the product of conductivity (κ) and the volume (V) of the solution containing one mole of the electrolyte.

Λ = κ × V

On dilution, conductivity decreases but volume containing one mole of an electrolyte increases. The increase in volume on dilution is much more than the decrease in conductivity. As a result molar conductivity increases with dilution.

The molar conductance of strong (HCl, KCl, KNO₃) as well as weak electrolytes (CH₃COOH, NH₄OH) increase with decrease in concentration or increase in dilution.

Equivalent conductivity also increases with dilution because of increase in volume containing one gram equivalent of the electrolyte.

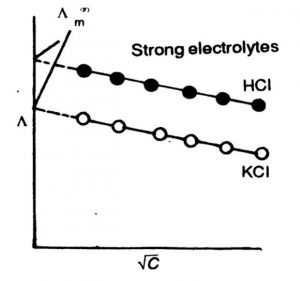
Variation of Molar Conductivity with Concentration for Strong and Weak Electrolytes

1) Variation of Molar Conductivity with Concentration for Strong Electrolytes

In strong electrolytes, molar conductivity increases slowly with dilution and there is a tendency for molar conductivity to approach a certain limiting value when the concentration approaches zero i.e., when the dilution is infinite. The molar conductivity when the concentration approaches zero (infinite dilution) is called molar conductivity at infinite dilution. It is denoted by Λ_m°

Λ_m = Λ_m°

when C——-> 0 (at infinite dilution)

The variation of molar conductivity with concentration may be given by the expression

Λ_m =  Λ_m° – AC^½

 

where A is a constant and Λ° is called molar conductivity at infinite dilution. This equation is called Debye Huckel Onsager equation and is found to hold good at low concentrations.

The variation of molar conductivity with concentration can be studied by plotting the values of Λ_m against square root of concentration (C^½).

The variation of Λ_m with concentration, C^½ is small so that the plots can be extrapolated to zero concentration. The intercept gives the limiting value of molar conductivity when the concentration approaches zero, called molar conductivity at infinite dilution, Λ_m. The slope of the line is equal to – ‘A’. The value of constant A for a given solvent and temperature depends on the type of electrolyte i.e., the charges on the cation anion produced on the dissociation of the electrolyte in the solution.

Thus NaCl is known as 1-1 electrolyte, CaCl₂ as 2-1 electrolytes and MgSO₄ as 2-2 electrolyte.

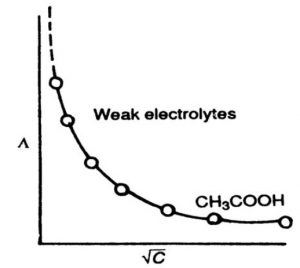
2) Variation of Molar Conductivity with Concentration for Weak Electrolytes

The weak electrolytes dissociate to a much lesser extent as compared to strrong electrolytes. Therefore, the molar conductivity is low as compare of strong electrolytes.

However, the variation of Λ_m  with C^½ is very large and so much so that we cannot obtain molar conductance at infinite dilution ( Λ_m°) by extrapolation of Λ_m versus C^½ plots.

Variation of Molar Conductivity with Concentration

 

a) Conductance behaviour of weak electrolytes

The number of ions furnished by an electrolyte in solution depends upon the degree of dissociation with dilution. With the increase in dilution, the degree of dissociation increases and as a result molar conductance increases. The limiting value of molar conductance (Λ_m) corresponds to degree of dissociation equal to 1, i.e. the whole of the electrolyte dissociates.

Thus, the degree of dissociation can be calculated at any concentration

α = Λ_m^c / Λ_m°

where α is the degree of dissociation, Λ_m^c  is the molar conductance at concentration C and Λ_m° is the molar conductance at infinite dilution.

b) Conductance behaviour of strong electrolytes

For strong electrolytes, there is no increase in the number of ions with dilution because strong electrolytes are completely ionised in solution at all concentrations.

In concentrated solutions of strong electrolytes there are strong forces of attraction between the ions of opposite charges called interionic forces. Due to these interionic forces the conducting ability of the ions is less in concentrated solutions. With dilution, the ions become far apart from one another and interionic forces decrease. As a result, molar conductivity increases with dilution. When the concentration of the solution becomes very very low, the interionic attractions become negligible and the molar conductance approaches the limiting value called molar conductance at infinite dilution.This value is characteristic of each electrolyte.

Kohlrausch’s Law

The difference of Λ° of different pairs of electrolytes having a common cation or a common anion was almost same.

Example 1 : the difference between the molar conductance of K⁺ and Na⁺ is 23.4 ohm^-1 cm² mol^-1 irrespective of the anion.

Λ° (KCl) = 149.9 

Λ° (NaCl) = 126.5 

Difference = Λ° (KCl) – Λ° (NaCl) = 149.9 – 126.5  = 23.4

Λ° (KNO₃) = 145

Λ° (NaNO₃) = 121.6 

Difference = Λ° (KNO₃) – Λ° (NaNO₃) = 145 – 121.6 = 23.4

Λ° (KBr) = 156.6 

Λ° (NaBr) = 128.2 

Difference =Λ° (KBr) – Λ° (NaBr) = 156.6 – 128.2  = 23.4

λ° (K⁺) – λ° (Na⁺) = 23.4 S cm² mol^-1

 

Example 2 :The difference between the molar conductivities of chloride and nitrate ions is 4.9  ohm^-1 cm² mol^-1 irrespective of the cation.

Λ° (KCl) = 149.9 

Λ° (KNO₃) = 145

Difference = Λ° (KCl) – Λ° (KNO₃) = 149.9 – 145 = 4.9 

Λ° (NaCl) = 126.5 

Λ° (NaNO₃) = 121.6

Difference = Λ° (NaCl) – Λ° (NaNO₃) = 126.5 -121.6 = 4.9

Λ° (LiCl) = 115

Λ° (NaNO₃) = 110.1 

Difference = Λ° (LiCl) – Λ° (NaNO₃) = 115 -110.1 = 4.9

λ° (Cl¯) – λ° (NO₃^–) = 4.9  S cm² mol^-1

Kohlrausch’s law states that, at infinite dilution when the dissociation is complete, each ion makes a definite contribution towards molar conductivity of the electrolyte irrespective of the nature of the other ion with which it is associated.

The molar conductivity at infinite dilution for a given salt can be expressed as the sum of the individual contributions from the ions of the electrolyte. If molar conductivity of the cation is denoted by λ°₊ and λ°_– then the law of independent migration of ions is :

Λ = ν₊ λ°₊ + ν_– λ°_–

where ν₊ and ν_– are the number of cations and anions per formula unit of electrolyte (e.g.= ν₊ = ν_– = 1  for HCl, ν₊= 1 and ν_– = 2 for MgCl₂).

λ°₊ and λ°_– are also  called molar ionic conductances at infinite dilution.

For example

1) For NaCl

Λ° (NaCl)= λ° (Na⁺) + λ° (Cl^–)

2) For KNO3

Λ° (KNO₃)= λ° (K⁺) + λ° (NO₃^–)

3) For Al₂(SO₄)₃

Λ° (Al₂(SO₄)₃)= 2λ° (Al³⁺) +3 λ° (SO₄^2-)

Applications of Kohlrausch’s Law

1) Calculation of Molar Conductance at Infinite Dilution for Weak Electrolytes

It is not possible to determine the value of limiting molar conductivity at infinite dilution for weak electrolytes by extrapolation of Λ versus C^½ graph. However this can be calculated easily by using Kohlrausch’s law.

The limiting molar conductivity for CH₃COOH. According to Kohlrausch’s law,

Λ°(CH₃COOH) = λ° (CH₃COO¯ ) + λ°(H⁺)

From Kohlrausch’s law,

Λ°(CH₃COONa) = λ° (CH₃COO¯ ) + λ°(Na⁺)

Λ° (HCl) = λ° (H⁺) + λ°(Cl‾)

Λ° (NaCl) = λ° (Na⁺) + λ°(Cl‾)

λ° (CH₃COO¯ ) + λ°(Na⁺) = [ (λ° (CH₃COO¯ ) + λ°(Na⁺))]+ [ λ° (H⁺) + λ°(Cl‾) ] – [ λ° (Na⁺) + λ°(Cl‾) ]

Λ°(CH₃COOH) = Λ°(CH₃COONa) + Λ° (HCl) – Λ° (NaCl)

 

2) Calculation of Degree of Dissociation of Weak Electrolytes

Molar conductance of a weak electrolyte depends upon its degree of dissociation. Higher the degree of dissociation, larger is the molar conductance.

With the increase in dilution, the conductance increases and at infinite dilution, the electrolyte is completely dissociated so that degree of dissociation becomes one i.e.

Λ = Λ° (at C—–> 0) Thus , if

Λ^c = molar conductance of solution at any concentration

Λ° = molar conductance at infinite dilution.

Then, degree of dissociation at any concentration is:

α = Λ^c /Λ°

Thus, measuring the molar conductance at any concentration (Λ^c ) helps to calculate degree of dissociation (α) if  is known.

3) Calculation of of Dissociation Constant of Weak Electrolytes

The dissociation constant (K) of weak electrolytes can be given as

K = Cα² / 1-α

where C is the concentration and α is the degree of dissociation.

Calculation of solubility product of sparingly soluble salts and Ionic Product of water

Salts such as AgCl, PbSO₄, BaSO₄ etc dissolve to a very small extent in water are called sparingly soluble salts.

Their solutions may be taken as infinite dilute. Moreover, because their solutions are saturated, their concentrations may be taken equal to their solubility. Thus, by determining the specific conductance (κ) and molar conductivity of such solutions, we can easily calculate solubility as:

Λ_m° = ( κ × 1000 ) / Molarity

Λ_m° = ( κ × 1000 ) / Solubility

Solubility = ( κ × 1000 ) / Λ_m°

Example: The specific conductance of a saturated solution of AgCl at 298 K is found to be 1.386 × 10^-6 S cm² mol^-1 . Calculate its solubility ( λ°_Ag⁺ = 62.0 S cm² mol^-1 and λ°_Cl¯ =76.3 S cm² mol^-1)

Λ°_m(AgCl) =  λ°(Ag⁺) + λ°(Cl¯)

Λ°_m(AgCl) = 62.0 + 76.3

Λ°_m(AgCl) =138.3 S cm² mol^-1

κ= 1.386 x 10^-6 S cm^-1

Solubility =( κ × 1000 ) / Λ°_m

Solubility = (1.386 × 10^-6  × 1000 ) / 138.3

Solubility = 1.435 × 10^-3 g L^-1

Ionic product of water can also be calculated by knowing the specific conductivity of water. 

At 298 K, specific conductivity of water, κ= 5.54 × 10^-8 S cm^-1

λ° (H⁺) = 349.6 S cm² mol^-1 

λ° (Cl¯) = 199.1  S cm² mol^-1 

Λ° (HCl) = λ° (H⁺) + λ° (Cl¯) =349.6 + 199.1  = 548.7  S cm² mol^-1

Λ_m° = ( κ × 1000 ) / Molarity

Molarity = ( κ × 1000 ) / Λ_m°

= (5.54 × 10^-8 × 1000 ) / 548.7

=1.01 × 10^-7 mol L^-1

Molarity = [H⁺] = [OH¯] = 1 × 10 ^-7

Ionic product of water , Kw = [H⁺]  [OH¯]

= (1.01 × 10^-7 ) (1.01 × 10^-7 )

= 1.02 × 10^-14