Contents
Pseudo Chemical Reactions
Some reactions are first order each with respect to two different reactants i.e.,
A+B——-> Products
Rate = k[A] [B]
If one of the reactants is present in high concentration (solvent) then there is very little change in its concentration. The concentration of that reactant remains practically constant during the reaction.
For example, if [A] = 0.01 M and that of solvent water [B] = 55.5 M, the concentration of B changes only from 55.50 to 55.49 M even after the completion of the reaction.
The reaction, therefore, behaves as a first order reaction in A. Such reactions are called pseudo first order reactions.
Consider the hydrolysis of ethyl acetate:
CH3COOC2H5 + H2O ⇔ CH3COOH + C2H5OH
The molecularity of the reaction is two because it involves two reacting species, namely ethyl acetate and water. However, the concentration of ethyl acetate changes during the reaction while water is present in such a large excess that its concentration remains practically unchanged. Therefore, the rate of the reaction depends only the order of the reaction is one.
Rate = k’ [CH3COOC2H5] [H2O]
on the concentration of ethyl acetate and hence
[H2O] can be takes as constant so that
Rate = k [CH3COOC2H5]
k= k’ [H2O]
Thus, the reaction appears to be second order but follows the first order kinetics. Such reactions which appear to be of higher order but actually follow lower order kinetics are called pseudo chemical reactions.
For example: The hydrolysis of cane sugar or inversion of cane sugar to give glucose
and fructose:
C12H22O11 + H2O ——–> C6H12O6 + C6H12O6
Molecularity is two while order is one.
Collision Theory
Collision theory was put forward by Max Trautz and William Lewis in 1916-18. It is based on kinetic theory of gases.
According to this theory,
1) the reactant molecules are assumed to be hard spheres and reaction is postulated to occur when molecules collide with each other.
2) The number of collisions that takes place per second per unit volume of the reaction mixture is known as collision frequency Z. The value of collision frequency is normally very high.
3) For instance, under ordinary conditions of temperature and pressure, in a gaseous system, the collision frequency of binary collisions is of the order of 1025 to 1028.
4) If all the collisions are effective in forming the products, the reactions must be completed in a very short time.
5) All the collisions among the reacting species at a temperature are not effective in bringing about the chemical reaction.
The collisions which actually produce the products and therefore, result in the chemical reactions are called effective collisions.
There are two important barriers to a reaction namely
(i) energy barrier (ii) orientation barrier
(i) Energy barrier: For the reacting species to make effective collisions, they should have sufficient energy to break the chemical bonds in the reacting molecules. The minimum amount of energy which the colliding molecules must possess is known as threshold energy. This means that only those collisions of reactants will give products which possess energies greater than threshold energy.
(ii) Orientation barrier: The colliding molecules should also have proper orientation so that the old bonds may break and new bonds are formed.
Consider the reaction
NO2 (g) + NO2 (g) ——> N2O4 (g)
During this reaction, the products molecules are formed only when the colliding molecules have proper orientation at the time of collisions. These are called effective collisions.
When the molecules do not have proper orientation at the time of collision, they result in ineffective collisions and do not form the products.
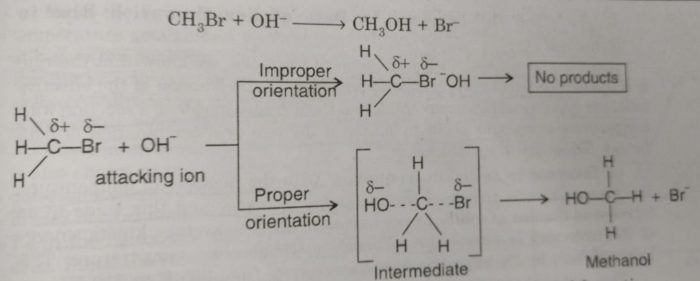
Consider the reaction of bromoethane with OH¯ ions to form methanol. The OH¯ ion must attack the positively charged carbon to form an intermediate which changes to product after elimination of Br¯ ion. If OH¯ does not get proper site for attack, reaction will not occur. First an intermediate is formed by the attack of OH‾ at the site away form Br¯ and finally product is formed as shown ahead :
Proper orientation of reactants leads to the bond formation whereas improper orientation simply makes them to bounce back and products are not formed.
Thus, the collisions in which the colliding molecules do not possess the minimum energy for effective collisions (threshold energy) or proper orientation do not form products. In other words, the colliding species rebound unchanged in such cases. Therefore, it follows that only a small fraction of collisions is effective.
Thus, the main points of collision theory are summed up below:
(i) For a reaction to occur, there must be collisions between the reacting species.
(ii) Only a certain fraction of the total number of collisions are effective in forming the products.
(iii) For effective collisions, the molecules should possess sufficient energy as well as orientation.
Thus, the rate of reaction is proportional to
(a) the number of collisions per unit volume per second (collision frequency, Z) between the reacting species,
(b) the fraction of effective collisions (properly oriented and possessing sufficient energy), f
Rate = -dx/dt = f × Z
Dependence Of Reaction Rates On Temperature
An increase in temperature increases the rate of almost all reactions. A decrease in temperature decreases the rate.
For example: the rate constant for the decomposition of N2O5 is 7.87 × 10-7 s-1 at 273 K but it becomes 3.56 x 10-5 s-1 at 298 K. This means that for a rise of 25° in temperature, the rate constant is increased by about 45 times.
In a mixture of potassium permanganate (KMnO4) and oxalic acid (H2C204), potassium permanganate gets decolourised faster at a higher temperature than at a lower temperature.
The rate of a reaction or rate constant becomes almost double for every 10° rise in temperature. This is also called temperature coefficient. It is the ratio of rate constants of the reaction at two temperatures differing by 10°.
Thus,
Explanation for Increase in Rate of Reaction with Rise in Temperature
According to collision theory of chemical reactions, the rate of a reaction depends upon collision frequency (Z) and fraction of the effective collisions (f).The increase in rate of reaction with temperature is brought about by either of the two or a combination of both factors.
These are discussed below :
we may think that the increase in rate of a reaction with
(i) Increase in collision frequency: With the increase in temperature, the average kinetic energy of the molecules increases and this leads to an increase in number of collisions per unit time (Z). The average kinetic energy of the molecules is directly proportional to the absolute temperature. Therefore, the increase in rate of a reaction is not simply due to the increase in collision frequency.
(ii) Effective collisions: According to the collision theory, only a small fraction of collisions is effective in bringing about the chemical reaction and the rest of the collisions are ineffective. For effective collision (to yield products) the colliding molecules must have more than or equal to certain minimum amount of energy called threshold energy. If the energy of the reacting molecules is less than this value, collisions will be ineffective.
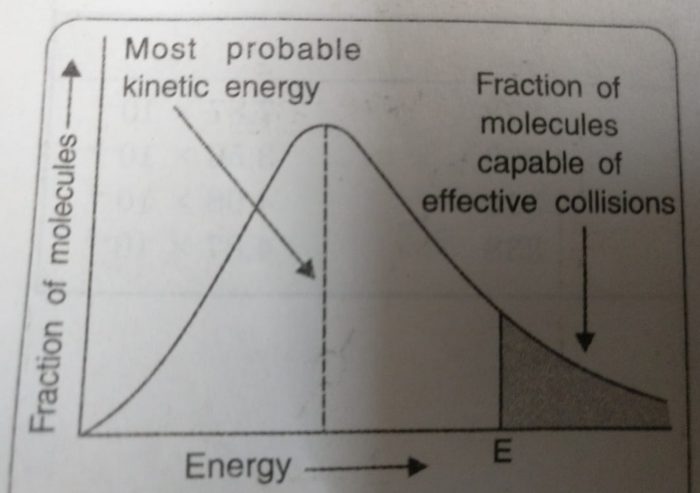
All the molecules in a substance do not possess the same kinetic energy. This is due to collisions between the moving molecules so that their energies are transferred from one molecule to another. Thus, there is a distribution of kinetic energies among reacting molecules. If the energy of molecules are plotted against the corresponding fraction of molecules, NE/NT with a given energy at a particular temperature, a curve is obtained. This is called Maxwell’s distribution of energies.
1) The fraction of molecules having very low or very high energies is very small.
2) Most of the molecules have intermediate kinetic energies as shown by the peak in the graph. This peak corresponds to most probable kinetic energy i.e. kinetic energy of maximum fraction of molecules.
3)E corresponds to minimum or threshold energy required for effective collisions. The molecules having energy equal to or greater than E will result in the formation of products and this fraction of molecules capable of effective collisions is very small.
4) For reactions having low values of E, there will be larger fraction of colliding molecules which produce effective collisions and hence, rate of the reaction will be high.
If the value of E for a particular reaction is high, then only a few collisions will be sufficiently energetic to give products while all other collisions will be ineffective. The reaction will proceed very slowly.
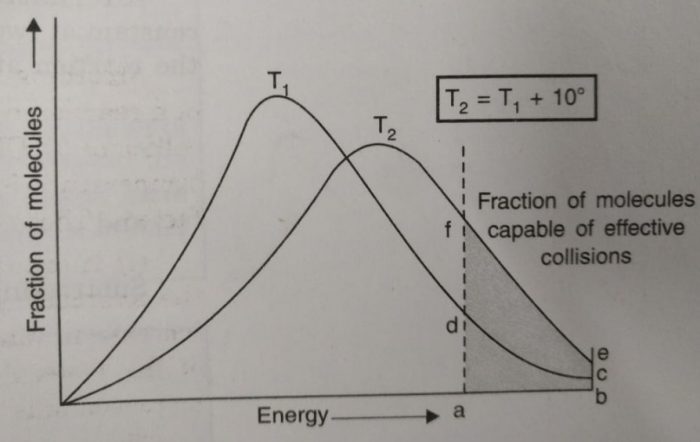
Effect of increase in temperature on the number of effective collisions
a) The curve at higher temperature gets shifted towards the right indicating that at higher temperature, the molecules have higher energies.
b) The curve at higher temperature is flatter than that at lower temperature which also indicates that the number of molecules with higher energy content have increased.
c) The number of molecules possessing energies equal to or greater than E, is proportional to area abcd at temperature T1, and area abef at temperature T2. The area abef is roughly twice as large as abcd.
Since the rate of reaction depends upon the number of molecules which possess energies larger than activation energy (for effective collisions), it may be interpreted that the fraction of molecules possessing activation energy has increased approximately two times and thereby, increases the rate by two times for a rise of 10 degrees.
Increase in the rate of reaction with the rise in temperature is mainly due to the increase in number of effective collisions.
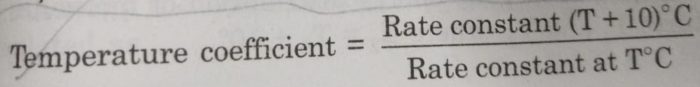
Nice explanation, very helpful for my project.
Thank you for this…