Exercise 6.4
Permutations and combinations
Question and Answers
Class 11 – Maths
| Class | Class 11 |
| Subject | Mathematics |
| Chapter Name | Permutations and Combinations |
| Chapter No. | Chapter 6 |
| Exercise | Exercise 6.4 |
| Category | Class 11 Maths NCERT Solutions |
Question 1 If nC8 = nC2, find nC2.
Answer nCa = nCb
⇒ a = b or n = a+ b
n = 8 + 2 =10
Therefore
Question 2 Determine n , if
(i) 2nC3 = nC3 = 12 : 1
⇒ 2n – 1 = 3(n – 2)
⇒ 2n – 1 = 3n – 6
⇒ 3n – 2n = -1 + 6
⇒ n = 5
(ii) 2nC3 = nC3 = 11 : 1
⇒ 4(2n – 1) = 11(n – 2)
⇒ 8n – 4 = 11n – 22
⇒ 11n – 8n = -4 + 22
⇒ 3n = 18
⇒ n = 6
Question 3 How many chords can be drawn through 21 points on a circle?
Answer For drawing one chord a circle, only 2 points are required.
To know the number of chords that can be drawn through the given 21 points on a circle, the number of combinations have to be counted.Therefore, the chords can be drawn through 21 points taken 2 as equal to each chord.
Thus, required number of chords =
⇒ 210
Question 4 In how many ways can a team of 3 boys and 3 girls be selected from 5 boys and 4 girls?
Answer A team of 3 boys and 3 girls is to be selected from 5 boys and 4 girls.
3 boys can be selected from 5 boys in 5C3 ways.
3 girls can be selected from 4 girls in 4C3 ways.
Number of ways in which a team of 3 boys and 3 girls can be selected =
⇒40
Question 5 Find the number of ways of selecting 9 balls from 6 red balls, 5 white balls and 5 blue balls if each selection consists of 3 balls of each colour.
Answer There are a total of 6 red balls, 5 white balls, and 5 blue balls.
9 balls have to be selected in such a way that each selection consists of 3 balls of each colour.
Here,
3 balls can be selected from 6 red balls in 6C3 ways.
3 balls can be selected from 5 white balls in 5C3 ways.
3 balls can be selected from 5 blue balls in 5C3 ways.
Required number of ways of selecting the 9 balls will be –
∴ The number of ways of selecting 9 balls from 6 red balls, 5 white balls and 5 blue balls if each selection consists of 3 balls of each colour is 6C3 ×5C3 × 5C3 = 2000
Question 6 Determine the number of 5 card combinations out of a deck of 52 cards if there is exactly one ace in each combination.
Answer We have a deck of 52 cards, which contains 4 aces. When we make a combination, 5 cards should be made in such a manner that there is exactly one ace.
Then, one ace can be selected in 4C1 ways and the remaining 4 cards can be selected out of the 48 cards in 48C4 ways.
The required number of 5 card combinations will be –
Question 7 In how many ways can one select a cricket team of eleven from 17 players in which only 5 players can bowl if each cricket team of 11 must include exactly 4 bowlers?
Answer The number of players out of which we have to select is 17 players and only 5 players from them are bowlers.
A cricket team of 11 players is to be selected in such a way that there are exactly 4 bowlers.
4 bowlers can be selected in 5C4 ways and the remaining 7 players can be selected out of the 12 players in 12C7 ways.
Required number of ways of selecting cricket team –
Question 8 A bag contains 5 black and 6 red balls. Determine the number of ways in which 2 black and 3 red balls can be selected.
Answer There are 5 black and 6 red balls in the bag.
2 black balls can be selected out of 5 black balls in 5C2 ways and 3 red balls can be selected out of 6 red balls in 6C3 ways.
Required number of ways of selecting 2 black and 3 red balls –
Question 9 In how many ways can a student choose a programme of 5 courses if 9 courses are available and 2 specific courses are compulsory for every student?
Answer There are 9 courses available out of which, 2 specific courses are compulsory for every student.
Therefore, every student has to choose 3 courses out of the remaining 7 courses. This can be chosen in 7C3 ways.
Thus, required number of ways of choosing the programme –
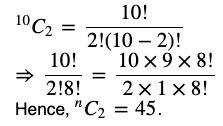
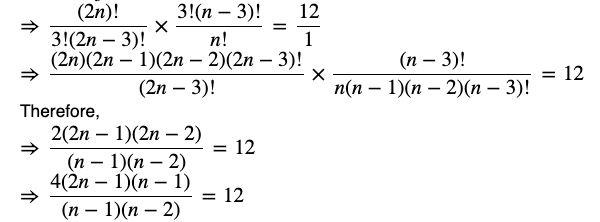
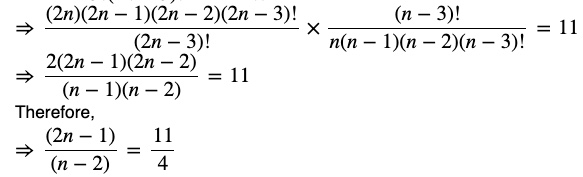
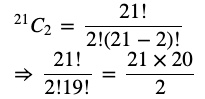
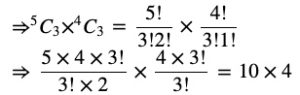
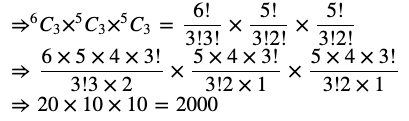
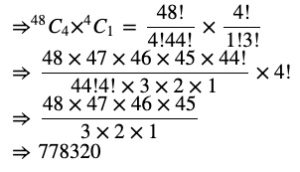
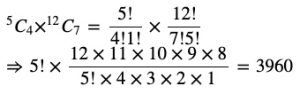
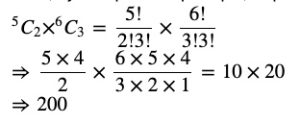
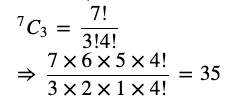
thanks mam for sharing free education