Exercise 5.1
Linear Equations
Question and Answers
Class 11 – Maths
| Class | Class 11 |
| Subject | Mathematics |
| Chapter Name | Linear Equations |
| Chapter No. | Chapter 5 |
| Exercise | Exercise 5.1 |
| Category | Class 11 Maths NCERT Solutions |
Question 1 Solve 24 x < 100, when
Answer The given inequality is 24x < 100

(i) x is a natural number.
(i) When x is a natural integer then the only natural number less than 25/6 are 1, 2, 3, 4.
Thus, 1, 2, 3, and 4 will be the solution of the given inequality when x is a natural number. Hence {1, 2, 3, 4} is the solution set.
(ii) x is an integer.
(ii) When x is an integer then the integer number less than 25/6 are…-1, 0, 1, 2, 3, 4.
Thus, solution of 24 x < 100 are…,-1, 0, 1, 2, 3, 4, when x is an integer. Hence {…, -1, 0, 1, 2, 3, 4} is the solution set.
Question 2 Solve – 12x > 30, when
Answer
The given inequality is – 12x > 30
(i) x is a natural number.
(i) When x is a natural integer then there is no natural number less than -2/5 because -5/2 is a negative number and natural numbers are positive numbers. Therefore there would be no solution of the given inequality when x is a natural number.
(ii) x is an integer.
(ii) When x is an integer then the integer number less than -5/2 are…, -5, -4, – 3.
Thus, solution of – 12x > 30 is …,-5, -4, -3, when x is an integer. Therefore the solution set is {…, -5, -4, -3}
Question 3 Solve 5x – 3 < 7, when
Answer Given that, 5x – 3 < 7
Now by adding 3 on both sides, we get,
5x – 3 + 3 < 7 + 3
The above inequality becomes
5 x < 10
Again, by dividing both sides by 5 we get,
5x/5 < 10/5
x < 2
(i) x is an integer
(i) The integers less than 2 are …, −4,−3,−2,−1,0,1 . As a result, the solutions to the above inequality are when is an integer…, −4,−3,−2,−1,0,1. As a result, the solution set in this example is {⋯,−4,−3,−2,−1,0,1}.
(ii) x is a real number.
When x is a real number, then the solutions of 5x – 3 < 7 will be given by x < 2 which states that all the real numbers that are less than 2. Hence the solution set is x ∈ (-∞, 2)
Question 4 Solve 3x + 8 >2, when
Answer Given that, 3x + 8 > 2
Now by subtracting 8 from both sides, we get,
3x + 8 – 8 > 2 – 8
The above inequality becomes,
3x > – 6
Again by dividing both sides by 3, we get,
3x/3 > -6/3
Hence x > -2
(i) x is an integer.
When x is an integer, then the integer numbers greater than -2 are -1, 0, 1, 2,…
Thus, solution of 3x + 8 > 2 is -1, 0, 1, 2,… when x is an integer.Hence the solution set is {-1, 0, 1, 2,…}
(ii) x is a real number.
When x is a real number. The solutions of 3x + 8 >2 will be given by x > -2 which means all the real numbers that are greater than -2. Therefore the solution set is x ∈ (-2, ∞).
Question 5 Solve the given inequality for real x : 4x + 3 < 5x+ 7
Answer
Given that, 4x + 3 < 5x + 7
Now by subtracting 7 from both the sides, we get
4x + 3 – 7 < 5x + 7 – 7
The above inequality becomes,
4x – 4 < 5x
Again, by subtracting 4x from both the sides,
4x – 4 – 4x < 5x – 4x
x > – 4
As a result, all real numbers x bigger than -4 are solutions to the given inequality. As a result, the given inequality’s solution set is (−4,∞).
Question 6 Solve the given inequality for real x : 3x – 7 > 5x – 1
Answer Given that, 3x – 7 > 5x – 1
Now, by adding 7 to both the sides, we get
3x – 7 +7 > 5x – 1 + 7
3x > 5x + 6
Again, by subtracting 5x from both the sides,
3x – 5x > 5x + 6 – 5x
-2x > 6
Dividing both sides by -2 to simplify, we get
-2x/-2 < 6/-2
x < -3
∴ The solutions of the given inequality are defined by all the real numbers less than -3. Hence the required solution set is (-∞, -3)
Question 7 Solve the given inequality for real x : 3(x – 1) ≤ 2 (x – 3)
Answer Given that, 3(x – 1) ≤ 2 (x – 3)
By multiplying, the above inequality can be written as
3x – 3 ≤ 2x – 6
Now, by adding 3 to both the sides, we get
3x – 3+ 3 ≤ 2x – 6+ 3
3x ≤ 2x – 3
Again, by subtracting 2x from both the sides,
3x – 2x ≤ 2x – 3 – 2x
x ≤ -3
Therefore, the solutions of the given inequality are defined by all the real numbers less than or equal to -3. Hence, the required solution set is (-∞, -3]
Question 8 Solve the given inequality for real x : 3 (2 – x) ≥ 2 (1 – x)
Answer Given that, 3 (2 – x) ≥ 2 (1 – x)
By multiplying, we get
6 – 3x ≥ 2 – 2x
Now, by adding 2x to both the sides,
6 – 3x + 2x ≥ 2 – 2x + 2x
6 – x ≥ 2
Again, by subtracting 6 from both the sides, we get
6 – x – 6 ≥ 2 – 6
– x ≥ – 4
Multiplying throughout inequality by negative sign, we get
x ≤ 4
∴ The solutions of the given inequality are defined by all the real numbers greater than or equal to 4. Hence the required solution set is (- ∞, 4]
Question 9 Solve the given inequality for real x : x + x/2 + x/3 < 11
Answer x + x/2 + x/3 < 11
The solutions of the given inequality are defined by all the real numbers less than 6. Hence the solution set is (-∞, 6)
Question 10 Solve the given inequality for real x : x/3 > x/2 + 1
Answer x/3 > x/2 + 1
– x / 6 > 1
-x > 6
x < -6
∴ The solutions of the given inequality are defined by all the real numbers less than – 6. Hence, the required solution set is (-∞, -6)
Question 11 Solve the given inequality for real x:
Answer
Now by cross-multiplying the denominators, we get
9(x- 2) ≤ 25 (2 – x)
9x – 18 ≤ 50 – 25x
Now adding 25x both the sides,
9x – 18 + 25x ≤ 50 – 25x + 25x
34x – 18 ≤ 50
Adding 25x both the sides,
34x – 18 + 18 ≤ 50 + 18
34x ≤ 68
Dividing both sides by 34,
34x/34 ≤ 68/34
x ≤ 2
The solutions of the given inequality are defined by all the real numbers less than or equal to 2.
Required solution set is (-∞, 2]
Question 12 Solve the given inequality for real x
Answer
Now by cross-multiplying the denominators, we get
∴ The solutions of the given inequality are defined by all the real numbers less than or equal to 120.Thus, (-∞, 120] is the required solution set.
Question 13 Solve the given inequality for real x : 2 (2x + 3) – 10 < 6 (x – 2)
Answer
2 (2x + 3) – 10 < 6 (x – 2)
By multiplying, we get
4x + 6 – 10 < 6x – 12
On simplifying, we get
4x – 4 < 6x – 12
4x – 6x < -12 + 4
-2x < -8
Dividing by 2, we get;
-x < -4
Multiply by “-1” and change the sign.
x > 4
∴ The solutions of the given inequality are defined by all the real numbers greater than 4.
Hence, the required solution set is (4, ∞).
Question 14 Solve the given inequality for real x : 37 – (3x + 5) ≥ 9x – 8 (x – 3)
Answer
Given that, 37 – (3x + 5) ≥ 9x – 8 (x – 3)
On simplifying, we get
= 37 – 3x – 5 ≥ 9x – 8x + 24
= 32 – 3x ≥ x + 24
On rearranging,
= 32 – 24 ≥ x + 3x
= 8 ≥ 4x
= 2 ≥ x
All the real numbers of x which are less than or equal to 2 are the solutions of the given inequalityHence, (-∞, 2] will be the solution for the given inequality.
Question 15 Solve the given inequality for real x :
Answer
On simplifying
All the real numbers of x which are greater than 4 are the solutions of the given inequality. Hence, (4, ∞) will be the solution for the given inequality.
Question 16 Solve the given inequality for real x :
Answer
= 20 (2x – 1) ≥ 3 (19x – 18)
= 40x – 20 ≥ 57x – 54
= – 20 + 54 ≥ 57x – 40x
= 34 ≥ 17x
= 2 ≥ x
∴ All the real numbers of x which are less than or equal to 2 are the solutions of the given inequality.Hence, (-∞, 2] will be the solution for the given inequality.
Question 17 Solve the given inequality and show the graph of the solution on number line: 3x – 2 < 2x + 1
Answer
3x – 2 < 2x + 1
Solving the given inequality, we get
3x – 2 < 2x + 1
= 3x – 2x < 1 + 2
= x < 3
Now, the graphical representation of the solution is as follows:
Question 18 Solve the given inequality and show the graph of the solution on number line: 5x – 3 ≥ 3x – 5
Answer
5x – 3 ≥ 3x – 5
Solving the given inequality, we get
5x – 3 ≥ 3x – 5
On rearranging, we get
= 5x – 3x ≥ -5 + 3
On simplifying,
= 2x ≥ -2
Now, dividing by 2 on both sides, we get
= x ≥ -1
The graphical representation of the solution is as follows:
Question 19 Solve the given inequality and show the graph of the solution on number line: 3 (1 – x) < 2 (x + 4)
Answer
3 (1 – x) < 2 (x + 4)
Solving the given inequality, we get
3 (1 – x) < 2 (x + 4)
Multiplying, we get
= 3 – 3x < 2x + 8
On rearranging, we get
= 3 – 8 < 2x + 3x
= – 5 < 5x
Now by dividing 5 on both sides, we get
-5/5 < 5x/5
= – 1 < x
Now, the graphical representation of the solution is as follows:
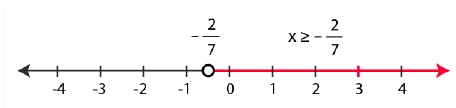
Question 20 Solve the given inequality and show the graph of the solution on number line:
Answer
Question 21 Ravi obtained 70 and 75 marks in the first two unit tests. Find the minimum marks he should get in the third test to have an average of at least 60 marks.
Answer
Let us assume that x is the marks obtained by Ravi in his third unit test.
According to the question, all the students should have an average of at least 60 marks
(70 + 75 + x)/3 ≥ 60
= 145 + x ≥ 180
= x ≥ 180 – 145
= x ≥ 35
Hence, all the students must obtain 35 marks in order to have an average of at least 60 marks.
Question 22 To receive Grade ‘A’ in a course, one must obtain an average of 90 marks or more in five examinations (each of 100 marks). If Sunita’s marks in first four examinations are 87, 92, 94 and 95, find minimum marks that Sunita must obtain in fifth examination to get grade ‘A’ in the course.
Answer
Let us assume Sunita scored x marks in her fifth examination
Now, according to the question, in order to receive A grade in the course, she must obtain an average of 90 marks or more in her five examinations
(87 + 92 + 94 + 95 + x)/5 ≥ 90
= (368 + x)/5 ≥ 90
= 368 + x ≥ 450
= x ≥ 450 – 368
= x ≥ 82
Hence, she must obtain 82 or more marks in her fifth examination
Question 23 Find all pairs of consecutive odd positive integers both of which are smaller than 10 such that their sum is more than 11.
Answer
Let us assume x to be the smaller of the two consecutive odd positive integers.
∴ The other integer is = x + 2
It is also given in the question that both the integers are smaller than 10.
∴ x + 2 < 10
x < 8 … (i)
Also, it is given in the question that the sum of two integers is more than 11.
∴ x + (x + 2) > 11
2x + 2 > 11
x > 9/2
x > 4.5 … (ii)
Thus, from (i) and (ii), we have,
x is an odd integer and it can take values 5 and 7.
Hence, possible pairs are (5, 7) and (7, 9)
Question 24 Find all pairs of consecutive even positive integers, both of which are larger than 5 such that their sum is less than 23.
Answer
Let us assume x is the smaller of the two consecutive even positive integers.
∴ The other integer = x + 2
It is also given in the question that both the integers are larger than 5.
∴ x > 5 ….(i)
Also, it is given in the question that the sum of two integers is less than 23.
∴ x + (x + 2) < 23
2x + 2 < 23
x < 21/2
x < 10.5 … (ii)
Thus, from (i) and (ii) we have x is an even number and it can take values 6, 8 and 10.
Hence, possible pairs are (6, 8), (8, 10) and (10, 12)
Question 25 The longest side of a triangle is 3 times the shortest side and the third side is 2 cm shorter than the longest side. If the perimeter of the triangle is at least 61 cm, find the minimum length of the shortest side.
Answer
Let us assume the length of the shortest side of the triangle to be x cm.
∴ According to the question, the length of the longest side = 3x cm
And, length of third side = (3x – 2) cm
As, the least perimeter of the triangle = 61 cm
Thus, x + 3x + (3x – 2) cm ≥ 61 cm
= 7x – 2 ≥ 61
= 7x ≥ 63
Now dividing by 7, we get
= 7x/7 ≥ 63/7
= x ≥ 9
Hence, the minimum length of the shortest side will be 9 cm.
Question 26 A man wants to cut three lengths from a single piece of board of length 91cm. The second length is to be 3cm longer than the shortest and the third length is to be twice as long as the shortest. What are the possible lengths of the shortest board if the third piece is to be at least 5cm longer than the second?
[Hint: If x is the length of the shortest board, then x , (x + 3) and 2x are the lengths of the second and third piece, respectively. Thus, x + (x + 3) + 2x ≤ 91 and 2x ≥ (x + 3) + 5].
Answer
Let us assume the length of the shortest piece to be x cm
∴ According to the question, length of the second piece = (x + 3) cm
And, length of third piece = 2x cm
As all the three lengths are to be cut from a single piece of board having a length of 91 cm
∴ x + (x + 3) + 2x ≤ 91 cm
= 4x + 3 ≤ 91
= 4x ≤ 88
= 4x/4 ≤ 88/4
= x ≤ 22 … (i)
Also, it is given in the question that, the third piece is at least 5 cm longer than the second piece.
∴ 2x ≥ (x+3) + 5
2x ≥ x + 8
x ≥ 8 … (ii)
Thus, from equation (i) and (ii), we have:
8 ≤ x ≤ 22
Hence, it is clear that the length of the shortest board is greater than or equal to 8 cm and less than or equal to 22 cm.
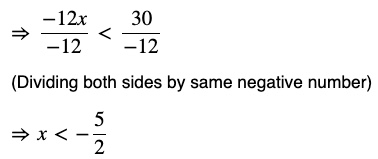
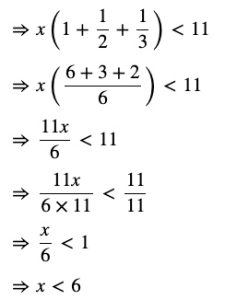

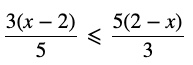
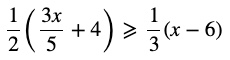
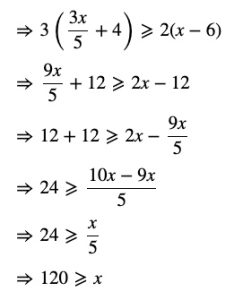
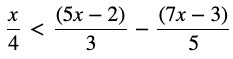
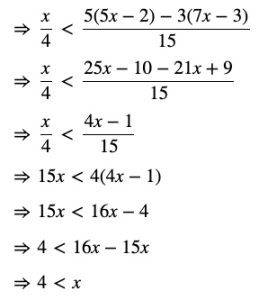
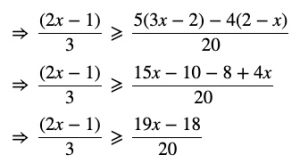
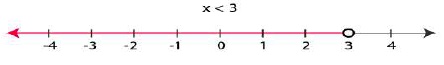
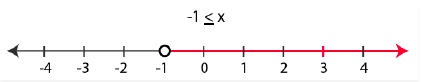
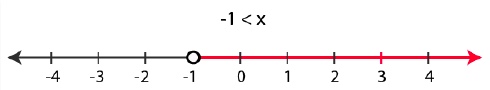
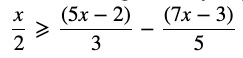
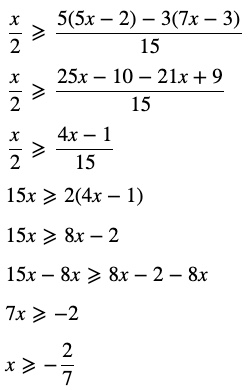
Leave a Reply